When we talk about how nuclear weapons work, we inevitably mention the “critical mass.” This is the amount of fissile material you need to create a self-sustaining nuclear reaction. But it’s a very tricky concept, one often poorly deployed and explained, and the result, I have found while teaching and while talking to people online, is an almost universal confusion about what it means on a physical level.
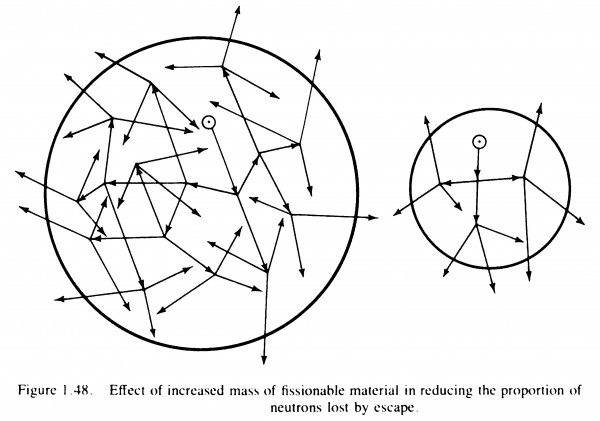
One of the ways in which the critical mass is visually explained in Glasstone and Dolan’s The Effects of Nuclear Weapons (1977 edn.). Want it on a t-shirt? I’ve got you covered.
Where does the term come from? In the Smyth Report released in August 1945, the term “critical size” is used almost universally, while “critical mass” is used exactly once (and parenthetically, at that). A more interesting term, the “critical condition,” is used in a few places. The Los Alamos Primer, from 1943, uses critical “radius,” “volume,” “conditions,” in addition to “mass.” The MAUD Report, from 1941, uses critical “size,” “value,” and “amount” — not mass. The Frisch-Peirels memorandum, from 1940, uses critical “radius,” “size,” and “condition.” Leo Szilard’s pre-fission, 1935 patent on chain reacting systems uses the terms critical “thickness,” and “value,” not mass. This is not to imply that people didn’t use the term “critical mass” at the time — but it was one term among many, not the only term. The earliest context I have found it being used extensively comes from a paper in 1941, where it was being used specifically to talk about whether masses of fissile material could be made to explode on demand and not before.1
Why use “critical mass” instead of other terms? For one thing, talking about the mass can help you get a sense of the size of the problem when fissile material is scarce and hard to produce (producing fissile material consumed 80% of the Manhattan Project’s budget). And it can also help you when talking about safety questions — about avoiding a nuclear reaction until you absolutely want on. So you don’t want to inadvertently create a critical mass. And knowing that the critical mass is so many kilograms of fissile material, as opposed to so many tons, was an early and important step in deciding that an atomic bomb was feasible in the first place.

A 5.3 kg ring of 99.96% pure plutonium. Under some conditions, this is enough to produce a significant explosive output. In its current form — unreflected, at normal density, in a ring-shape that prevents any neutrons from finding too many atoms to fission with — it is relatively innocuous.
What I don’t like about the term, though, is that it can easily lead to confusion. I have seen people assert, for example, that you need a “critical mass” of uranium-235 to start a nuclear reaction. Well, you do — but there is no one critical mass of uranium-235. In other words, used sloppily, people seem to often think that uranium-235 or plutonium have single values for their “critical mass,” and that “a critical mass” of material is what you use to make a bomb. But it’s more complicated than that, and this is where I think focusing on the mass can lead people astray.
Put simply, the amount of fissile material you need to start a nuclear reaction varies by the conditions under which it is being considered. The mass of material matters, but only if you specify the conditions under which it is being kept. Because under different conditions, any given form of fissile material will have different critical masses.
I’ve seen people (mostly online) want to talk about how nuclear weapons work, and they look up what “the” critical mass of uranium-235 is, and they find a number like 50 kg. They then say, OK, you must need 50 kg to start a nuclear reaction. But this is wrong. 50 kg of uranium-235 is the bare sphere critical mass of uranium-235. In other words, if you assembled 50 kg of uranium-235 into a solid sphere, with nothing around it, at normal atmospheric conditions, it will start a self-sustaining chain reaction. It probably would not produce an explosion of great violence — the uranium sphere would probably just blow itself a few feet apart (and irradiate anyone nearby). But once blown apart, the reaction would stop. Not a bomb.

The Godiva Device, a “naked” (get it?) critical assembly used as a pulsed nuclear reactor at Los Alamos. A 54 kg near-bare sphere of 93.7% enriched uranium separated into three pieces. At left, it is separated safely — no reaction. At right, you see what happened when the pieces got close enough to start a critical reaction — not a massive explosion (thank goodness), but enough energy output to damage the machine, and to push those pieces of uranium far enough from each other that they could no longer react. The workers were fortunately a safe distance away.
So does that mean that 50 kg of uranium-235 is a important number in and of itself? Only if you are assembling solid spheres of uranium-235.
Is 50 kg the amount you need for a bomb? No. You can get away with much smaller numbers if you change the conditions. So if you put a heavy, neutron-reflecting tamper around the uranium, you can get away with around 10 to 15 kg of uranium-235 for a bomb — a factor of 3-5X less mass than you thought you needed. If your uranium-235 is dissolved in water, it takes very low masses to start a self-sustaining reaction — a dangerous condition if you didn’t mean to start one! And it may be possible, under very carefully-developed conditions, to make a bomb with even smaller masses. (The bare-sphere critical mass of plutonium is around 10 kg, but apparently one can get a pretty good bang out of 3-4 kg of it, if not less, if you know what you are doing.)2
Conversely, does this mean that you can’t possibly have 50 kg of uranium (or more) in one place without it detonating? No. If your uranium is fashioned not into a solid sphere, but a cylinder, or is a hollow sphere, or has neutron-absorbing elements (i.e. boron) embedded in it, then you can (if you know what you are doing) exceed that 50 kg number without it reacting. And, of course, there are also impurities — the amount of uranium-238 in your uranium-235 will increase the size of any critical mass calculation.
In other words, under different conditions, the mass of fissile material that will react varies, and varies dramatically. These different conditions include different geometries, densities, temperatures, chemical compositions/phases, and questions about whether it is embedded into other types of materials, whether there are neutron-moderating substances (i.e. water) present, enrichment levels, and so on. It’s not a fixed number, unless you also fix all of your assumptions about the conditions under which it is taken place.

Re-creation of Louis Slotin’s fatal experiment with the third plutonium core. The problem wasn’t the mass of the core, it was that Slotin inadvertently changed the state of the system (by accidentally letting the reflector drop onto it completely when his screwdriver slipped), which took a safe, non-critical assemble of plutonium and moved it into a briefly-critical state. This produced no explosion, but enough radiation to be fatal to Slotin and damaging to others in the room.
The classic example of this, of course, is the implosion bomb design. The bare sphere critical mass of plutonium-239 is 10 kg. The Nagasaki bomb contained 6.2 kg of plutonium as its fuel. At normal, room-temperature densities, a solid sphere of 6.2 kg of plutonium is not critical. Increase its density by 2.5X through the careful application of high explosives, however, and suddenly that is at least one critical mass of plutonium. Even this is something of an oversimplification, because it’s not just the density that matters: the allotropic (chemical) phase of plutonium, for example, affects its critical mass conditions (and plutonium is notorious for having an unusual number of these phases), and the Nagasaki bomb also included many other useful features meant to help the reaction along like a neutron initiator (which gave it a little shot of about 100 neutrons to start things off), and a heavy, natural-uranium tamper.
What I dislike about the term “critical mass,” as well, is that it can serve to obscure the physical process that defines “criticality.” It can make it seem like reactivity is a function of the mass alone, which is wrong. Worse, it can keep people from realizing why the mass matters in the way it does (among other things). And this can lead to confusion on questions like, “how much explosive power does a critical mass release?” The answer is… it has nothing to do with the critical mass per se. That is a question of bomb efficiency, which can seem like a secondary, separate question. But both the question of criticality and efficiency are really one and the same phenomena — if you understand the underlying physical process on an intuitive level.
Criticality, the “critical condition,” is defined as the point at which a chain reacting system becomes self-sustaining. So we can imagine a whole sea of uranium-235 atoms. Neutrons enter the system (either from a neutron source, spontaneous fissioning, or the outside world). If they are absorbed by a uranium-235 nucleus, they have a chance of making it undergo fission. That fission reaction will produce a random number (2.5 on average) of secondary neutrons. To be critical, enough of these neutrons will then have to go on to find other uranium nuclei to keep the overall level of neutrons (the “neutron economy”) constant. If that total number of neutrons is very low, then this isn’t very interesting — one neutron being replenished repeatedly isn’t going to do anything interesting. If we’ve already got a lot of neutrons in there, this will generate a lot of energy, which is essentially how a nuclear reactor works once it is up and running.
Supercriticality, which is what is more important for bomb design (and the initial stages of running a reactor) is when your system produces more than one extra neutron in each generation of fissioning. So if our uranium atom splits, produces 2 neutrons, and each of those go on to split more atoms, we’re talking about getting two neutrons for every one we put into the system. This is an exponentially-growing number of neutrons. Since neutrons move very quickly, and each reaction takes place very quickly (on the order of a nanosecond), this becomes a very large number of neutrons very quickly. Such is a bomb: an exponential chain reaction that goes through enough reactions very quickly to release a lot of energy.

A sectional view of a rendering of the Trinity “Gadget” I made. The 6.2 kg sphere of plutonium (the second-to-last sphere in the center, which encloses the small neutron initiator) is a safe-to-handle quantity by itself, and only has the possibility of becoming super-critical when the high explosives compress it to over twice its original density. Sizes are to scale.
So what are the conditions that produce these results? Well, it’s true that if you pure enough fissile material in one place, in the right shape, under the right conditions, it’ll become critical. Which is to say, each neutron that goes into the material will get replaced by at least another neutron. It will be a self-sustaining reaction, which is all that “criticality” means. Each fission reaction produces on average 2.5 more neutrons, but depending on the setup of the system, most or all of those may not find another fissile nuclei to interact with. If, however, the system is set up in a way that means that the replacement rate is more than one neutron — if every neutron that enters or is created ends up creating in turn at least two neutrons — then you have a supercritical system, with an exponentially-increasing number of neutrons. This is what can lead to explosions, as opposed to just generating heat.
In a bomb, you need more than just a critical reaction. You need it to be supercritical, and to stay supercritical long-enough that a lot of energy is released. This is where the concept of efficiency comes into play. In theory, the Fat Man’s 6.2 kg of plutonium could have released over 100 kilotons worth of energy. In practice, only about a kilogram of it reacted before the explosive power of the reaction separated the plutonium by enough that no more reactions could take place, and “only” released 20 kilotons worth of energy. So it was about 18% efficient. The relative crudity of the Little Boy bomb meant that only about 1% of its fissile material reacted — it was many times less efficient, even though it had roughly 10X more fissile material in it than the Fat Man bomb. The concept of the critical mass, here, really doesn’t illuminate these differences, but an understanding of how the critical reactions work, and how the overall system is set up, does.
This understanding of criticality is more nuanced than a mere mass or radius or volume. So I prefer the alternative phrasing that was also used by weapons designers: “critical assembly” or “critical system.” Because that emphasizes that it’s more than one simple physical property — it’s about how a lot of physical properties, in combination with engineering artifice, come together to produce a specific outcome.
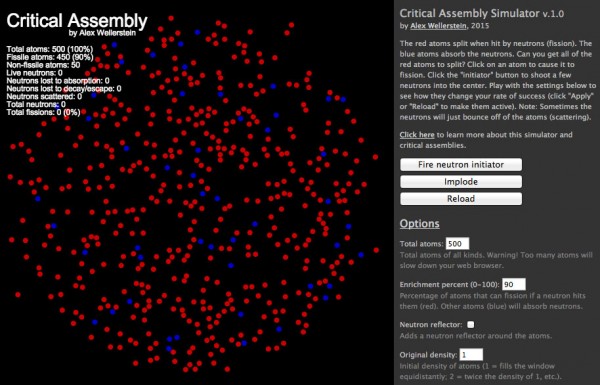
I’ve been playing around with the scripting language Processing.js recently, in my endless quest to make sure my web and visualization skills are up-to-date. Processing.js is a language that makes physics visualizations (among other things) pretty easy. It is basically similar to Javascript, but takes care of the “back end” of graphics to a degree that you can just say, “create an object called an atom at points x and y; render it as a red circle; when it comes into contact with another object called a neutron, make it split and release more neutrons,” and so on. Obviously it is a little more arcane than just that, but if you have experience programming, that is more or less how it works. Anyway, I had the idea earlier this week that it would be pretty easy to make a simple critical assembly “toy” simulation using Processing, and this is what I produced:
The gist of this application is that the red atoms are uranium-235 (or plutonium), and the blue atoms are uranium-238 (or some other neutron-absorbing substance). Clicking on an atom will cause it to fission, and clicking on the “fire neutron initiator” button will inject a number of neutrons into the center of the arrangement. If a neutron hits a red atom, it has a chance to cause it to fission (and a chance to just bounce off), which releases more atoms (and also pushes nearby atoms away). If it hits a blue atom, it has a chance to be absorbed (turning it purple).
The goal, if one can put it that way, is to cause a chain reaction that will fission all of the atoms. As you will see from clicking on it, in its initial condition it is hard to do that. But you can manipulate a whole host of variables using the menu at the right, including adding a neutron reflector, changing the number of atoms and their initial packing density, the maximum number of neutrons released by the fission reaction, and even, if you care to, changing things like the lifetimes of the neutrons, the likelihood of the neutrons just scattering off of atoms, and whether the atoms will spontaneously fission or not. If you have a reflector added, you can also click the “Implode” button to make it compress the atoms into a higher density.3
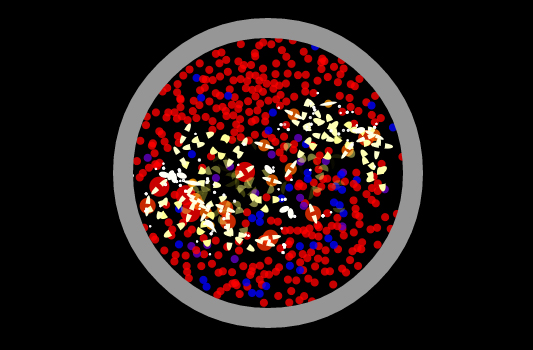
The progress of a successful reaction using an imploded reflector. The little yellow parts are a “splitting atom” animation which is disabled by default (because it decreases performance).
This is not a real physical simulation of a bomb, obviously. None of the numbers used have any physically-realistic quality to them, and real atomic bombs rely on the fissioning of trillions of atoms in a 3D space (whereas if you try to increase the number of atoms visible to 1,000, much less 10,000, your browser will probably slow to a crawl, and this is just in 2D space!).4 And this simulator does not take into account the effects of fission products, among other things. But I like that it emphasizes that it’s not just the number of atoms that determines whether the system is critical — it’s not just the mass. It’s all of the other things in the system as well. Some of them are physical constants, things pertaining to the nature of the atoms themselves. (Many of these were constants not fully known or understood until well after 1939, which is why many scientists were skeptical that nuclear weapons were possible to build, even in theory.) Some of them are engineering tricks, like the reflector and implosion.
My hope is that this kind of visualization will help my students (and others) think through the actual reaction itself a bit more, to help build an intuitive understanding of what is going on, as a remedy to the aspects of a prior language that was created by scientists, diffused publicly, and then got somewhat confused. “Critical mass” isn’t a terrible term. It has its applications. But when it can lead to easy misunderstandings, the language we choose to use matters.
- E.g. “Can system be controlled safely by dividing mass into two parts? Yes. We believe that it is possible with suitable technical supervision to assemble masses which will be known fractions of the critical mass and which will not explode during the assembly.” The authorship of the report is apparently several members of the Uranium Committee, but their specific names are unlisted. “Fast neutron chain reactions — Summary of discussion on recommendations of the Sub-section on theoretical aspects on October 24, 1941,” (24 October 1941), copy in Bush-Conant File Relating the Development of the Atomic Bomb, 1940-1945, Records of the Office of Scientific Research and Development, RG 227, microfilm publication M1392, National Archives and Records Administration, Washington, D.C., n.d. (ca. 1990), Reel 10, Target 21, Folder 162A, “Reports — Chain Reactions [1941].” [↩]
- On the “how low can you go” question, I have found table A.1 in this report useful:International Panel on Fissile Material, “Global Fissile Material Report 2013: Increasing Transparency of Nuclear Warhead and Fissile Material Stocks as a Step toward Disarmament,” Seventh annual report of the International Panel on Fissile Material (October 2013). There is documentary evidence suggesting the Soviets managed to weapons with cores as little as 0.8 kg of plutonium, and got significant (e.g. >1 kiloton) yields from them. [↩]
- For those who want it, the source code is here. It is sparsely commented. It is written, again, in Processing.js. [↩]
- Just to put this into perspective, 1 kg of plutonium-239 is ~2.5 x 1024 atoms. [↩]



This is an issue that confused me quite a bit before I finally “sat down with it” and got everything straight in my head. A big part of the issue is semantic or rhetorical. You got at this a little bit in talking about criticality vs. supercriticality, but the fact that you can talk about criticality in both a bomb and a reactor with very different consequences in each case can be very confusing.
When a reactor “goes critical” it means that the reactor is producing a self-sustaining reaction, but it sounds like the reactor is going to explode. After all, if a patient is in “critical condition,” it basically means the situation is “really bad”. The fact that both a reactor and a bomb can go critical probably has some contributing effect to the notion that if a reactor melts down or explodes, there is a danger of a bomb-style effect.
You can even be supercritical in a reactor (they usually are on startup, to get the neutron economy started). Yeah, I think it is a tricky word to use. I gets its historical origins, used by technical experts, but its migration into colloquial speech leaves a lot to be desired.
[[Alex surely already knows this, but others may not]]
On the “how low can you go?” question, Cochran and Paine wrote a classic 1995 NRDC report arguing that with a suitably high-tech implosion system, 1kg of Pu or 2.5kg of U-235 could produce a kiloton yield.
I believe it. The efficiency calculation is easy to do, as an aside — since you get around 18 kt of explosive output from every kilogram of fission, you can say that a 1 kg bomb that produced 1 kt would have to have about 0.06 kg of its mass fission, or 6% efficiency. For 2.5 kg, you only need 2% efficiency for the same output. That seems low (Fat Man had an efficiency of 17% or so), but getting something like that going in such a small mass is probably a non-trivial effort.
I’ve thought about this in the context of people asking “how small is the smallest theoretical nuclear weapon?” The Davy Crockett was the smallest American weapon fielded, but there is a difference between the smallest theoretical weapon and the smallest field-able weapon (because a fancy implosion system may not make for a good field weapon). I have not found anything that makes me think there is a theoretical low-end, if you are talking about laboratory conditions (it ends up scraping up against whatever definition we would have a “nuclear weapon” at that stage — with the bare minimum being something like the Slotin criticality accident, a weapon with no explosive output but a deadly neutron flux). Just practical difficulties that make it not worth it, along with economic considerations.
Carey Sublette wrote about the subject here:
http://nuclearweaponarchive.org/News/DoSuitcaseNukesExist.html
I played around with the simulator a bit. Imploding and then using an initiator vs. imploding and clicking on one atom didn’t necessarily get me a greater number of total fissions either way. Is that expected? Are initiators solely to ensure the reactions start, no questions?
In actual bombs, the initiators are both to make sure it starts and, I believe, also to give the reaction a head-start. It allows you to “skip ahead” several fission generations in terms of neutron economy. (If each generation of fissioning releases 2 neutrons, then 100 neutrons is the equivalent of between 6 or 7 generations of fissioning already occurring. There are only 50-80 generations in a kiloton-range weapon’s reaction, so that’s a nice jump, assuming they all react.) In the simulator, the initiator only produces 5 neutrons by default, so it is only a tiny bit better than the default settings for a single fissioning (which releases between 1 and 3 neutrons). The initiator in the Fat Man bomb produced around 100 neutrons, so not a huge number, but enough to make sure that it started when it was supposed to. I think later initiators probably could produce more neutrons than that.
Reed, on “The physics of the manhattan project” give an efficiency equation the also depends on the number of neutron at the beginning of the reaction. I can’t remeber it now (I’ll search for it tomorrow), but I remeber he wrote that it doesn’t make a very big difference in the efficiency.
Nice !
Seems like that Processing scripting language might be worth a look (I have no experience with Java programming).
In particular, I am interested in producing an interactive applet that generates contour plots of neutron multiplication factor (“k-infinity”) for heterogeneous lattices, based on a simple two-group set of formulas (referencing Bessel functions).
The graph has fuel channel diameters on one axis and lattice spacing on the other.
Decades ago, when we ran this program on a mainframe at university, the plotting of the numerical results had to be done by hand, which of course was extremely tedious if one wanted even just 3 or 4 contours.
I think that such an interactive applet could be very educational for students, particularly if it allowed input for a variety of fuel types (including molten fluoride salts), moderator types, and fuel channel wall material types.
This is what the results looked like, when plotted by hand:
https://dl.dropboxusercontent.com/u/11686324/HETERO_results_traced.jpg
As regards fission bombs, another visualization that might be of interest is one that illustrates how converging shockwave generators work.
In this respect, I made a little gif animation that shows how a “thermal bridge” disrupts a converging shockwave and interferes with effective implosion of a fissile pit. If you recall, the thermal bridge has been suggested as a way to address the issue of hot reactor-grade Pu. This gif animation is intended to show that it’s unlikely to succeed in making such material useful for weapons…..
https://dl.dropboxusercontent.com/u/11686324/Marks_RGPu_dud.gif
Cheers,
Jaro
Critical Assembly is fun. A modern way to visualize a nuclear chain reaction.
The old atomic energy museum in Oak Ridge, Tennessee had a cartoon that covered the same material, with the added bonus of explaining how to control the process for more peaceful uses.
On the subject of critical mass, it is interesting to compare Little Boy with Ivy King.
Both used much the same mass of U235, about 60 or so Kg. Taking Little Boy as 80% enrichment, the ratio of the product of critical radius and density to actual radius and density was about 1.4. Assuming a compression of about 2, then Ivy King was about 2.4. But more importantly was the expansion distance to 2nd criticality and the time scale of this. For Little Boy these are calculated at 1.7 cm and 32 ns and with a total mass of about 380 Kg gives a yield of 13500 tons TNT.
The figures for Ivy King are 4 cm and about 12 ns which assuming a similar mass gives a yield of the order of 420 K tons. It was reckoned that the uranium tamper contributed some thing like 20% to the yield.
A different design gave a bigger bang !
I love the Critical Assembly Simulator. I would sure like to have an offline version. Any chance of a link to source code for us GNU/Linux tinkerers?
Hi Richard — I’ve linked to the source code in a footnote to the post above. Here’s the link again. It can run offline (cross-platform) in the Processing environment.
Excellent article and visualizer. Great work, Alex!
This post and the discussion of initiators made me go back and look up a rather interesting article on a subminiature neutron generator developed by Sandia Labs and announced in 2012:
http://www.rdmag.com/award-winners/2012/08/first-ever-solid-state-neutron-generator
If a small slug (250 g ?) of U235 were highly compressed and then flooded with neutrons from an array of these small devices, would there be significant yield? Enough to drive an EMP generator or gamma laser? Could this explain the announcements of “subcritical” tests that appear in the open literature from time to time?